A quasi-meshfree method for nonlinear solid mechanics: Separating domain discretization from solution discretization

Abstract

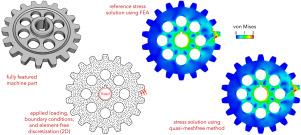
In many applications, domains of interest are geometrically complex containing numerous small features. These features are typically removed in a manual process to facilitate a conventional element-based meshing process. This manual defeaturing process is dependent upon the goals of the simulation and typically involves subjective heuristics. To provide a flexible and easily adaptable discretization process of the governing equations that is independent of the domain discretization, an element-free Galerkin method is proposed in which a fine-scale triangulation is used to first discretize the fully featured domain, but then a coarse-scale element-free discretization is used to approximate the solution of the governing equations. The fine-scale triangulation can be of poor quality and extremely refined since it is not used directly to approximate the solution of the governing equations. The coarse-scale element-free basis has local support and can be adapted through refinement or coarsening without the need to alter the fine-scale triangulation or other geometric considerations. The element-free basis functions are constructed using a conventional moving-least-squares procedure, but the initial weight functions are constructed using manifold geodesics for general applicability to non-convex domains. The weak form of the governing equations is integrated using a secondary coarse-scale element-free basis and a gradient projection technique. The projected-gradient methodology ensures the necessary consistency properties to pass the patch test and obtain optimal rates of convergence. The overall method is termed quasi-meshfree since both meshfree and mesh-based concepts are used. Several verification problems and nonlinear application examples are presented to demonstrate the overall method.